In a recent article titled “More transmissible versus more Lethal”, Nana Dadzie Ghansah correctly observed that:
“More transmissible is more dangerous than more lethal. A very transmissible but less lethal disease like COVID-19 will kill more people than a deadlier but less transmissible disease like Ebola. Prof. Adam Kucharski of the London School of Hygiene and Tropical Medicine puts it best in the attached model.”
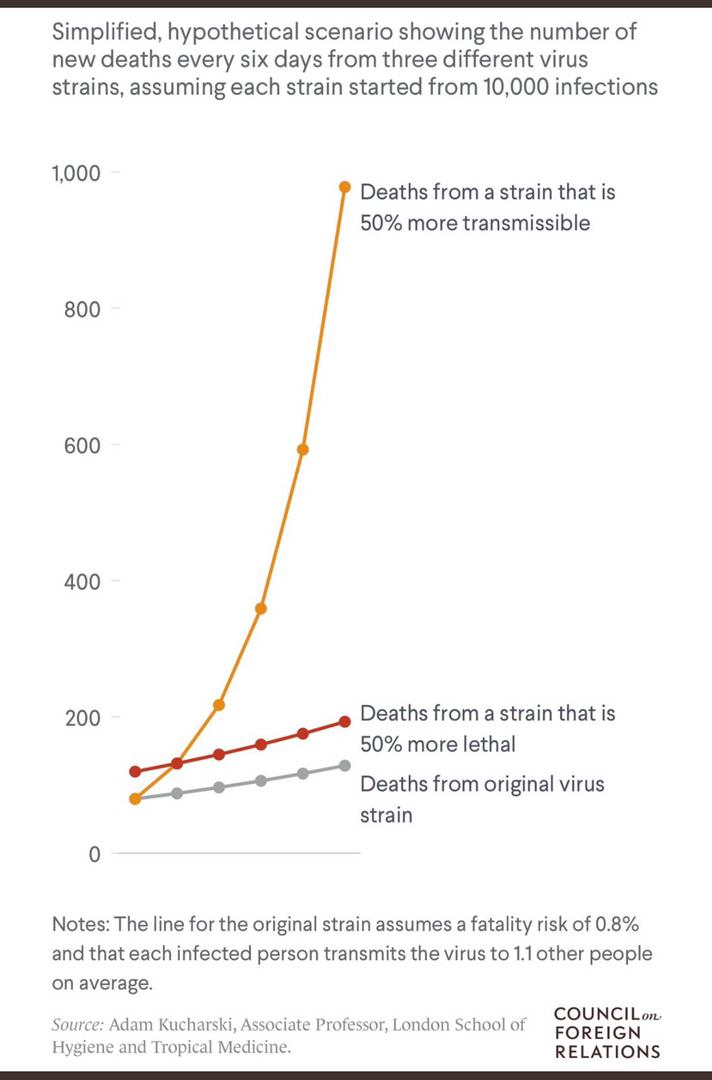
This accords with intuition but it may not be obvious. In particular, Professor Adam Kucharski (see diagram) demonstrated that a 50% increase in transmissibility results in more deaths than a 50% increase in lethality. Why is it that a 50% increase in each case has different effects?
Professor Kucharski assumed that 10,000 were initially infected. The rate of transmission was 1.1 per infected person. That is, an infected person can infect 1.1 persons. He also assumed that the rate of fatality (lethality) was 0.8%.
Case 1: Hold the rate of lethality constant at 0.8% and increase the rate of transmission by 50% (double) from 1.1 to 2.2. If an infected person dies, he infects others before his death.
Period 0 (initial period): 10,000 originally infected people; 2.2 times 10,000 = 22,000 new infections; 0.8% of original 10,000 = 80 people dead.
Period 1: 22,000 people were infected in period 0; 2.2 times 22,000 = 48,400 new infections; 0.8% of 22,000 = 176 people dead.
Period 2: 48,400 people were infected in period 1; 2.2 times 48,400 = 106,480 new infections; 0.8% of 48,400 = 387.2 people dead.
Total number of deaths = 80 + 176 + 387.2 = 643.2.
Case 2: Hold the rate of transmission constant at 1.1 and double the rate of lethality from 0.8% to 1.6%.
Period 0 (initial period): 10,000 originally infected people; 1.1 times 10,000 = 11,000 new infections; 1.6% of original 10,000 = 160 people dead.
Period 1: 11,000 people were infected in period 0; 1.1 times 11,000 = 12,100 new infections; 1.6% of 11,000 = 176 people dead.
Period 2: 12,100 people were infected in period 1; 1.1 times 12,100 = 13,310 new infections; 1.6% of 12,100 = 193.6 people dead.
Total number of deaths = 160 + 176 + 193.6 = 529.6.
These numerical examples show that a 50% increase in transmission results in more deaths than a 50% increase in fatality (i.e., 643.2 > 529.6). Consistent with professor Kucharski’s diagram, the number of deaths in periods 0 and 1 is higher when the lethality rate is higher. But in periods 2 and beyond, the number of deaths increases at a much faster rate when the rate of transmission is higher. This explains why it is incorrect for some people to argue that Covid-19 is like the flu because they both have low or comparable death rates. One must also take the rate of transmission into account. Covid-19 has a much higher transmission rate. One reason is that, unlike the flu, infected people who are asymptomatic can also infect others.
Infections lead to more infections and result in exponential growth rates. Infections build on each other from period to period. In contrast, deaths do not build on each other. Those who die leave the population. It does not have the dynamism of exponential growth. This explains why transmissibility is more dangerous than lethality. In terms number of deaths, hospitalization, and the impact on health facilities, etc, Covid-19 is not like the flu.
In general, suppose R is the transmission rate and D is the death (lethality) rate. Then given an initial population of 10,000 infected persons, it can be shown that in period T, the number of deaths is 10,000DR^T (10,000 times D times R raised to the power T). In any period T > 1, the rate of transmission, R, enters the formula for the number of deaths as an exponential term while the rate of lethality enters as a non-exponential term. There you have it. Higher transmissibility is, in general, more dangerous than higher lethality.
By J. Atsu Amegashie
January 17, 2021